Yunyun Chen, Chengxing He, Weihao Cheng, Wenzhuo Xie, "Phase unwrapping by a multi-level grid method for moiré fringes," Chin. Opt. Lett. 23, 021101 (2025)
- Chinese Optics Letters
- Vol. 23, Issue 2, 021101 (2025)
Fig. 1. Key principle of the method.
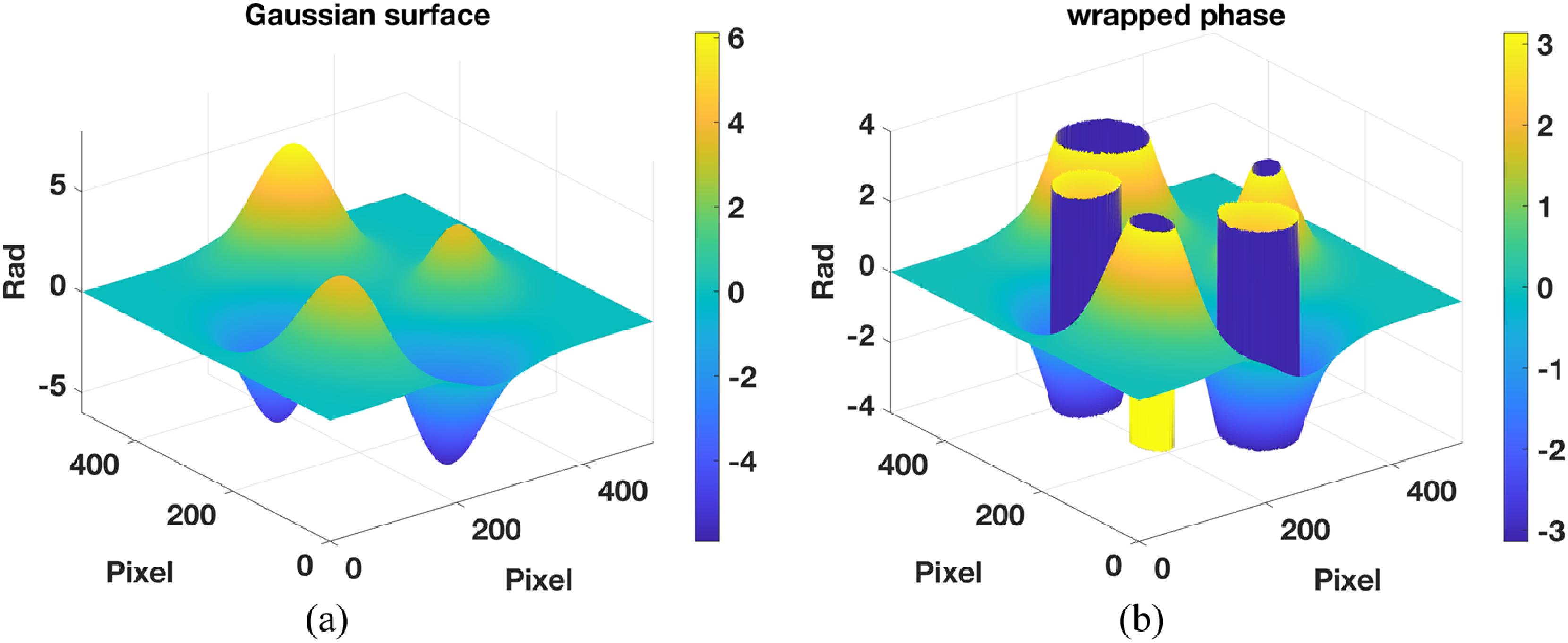
Fig. 2. A numerical simulation. (a) Gaussian surface; (b) wrapped phase.
Fig. 3. Phase unwrapping and errors: (a) multi-level grid method (proposed in this paper); (b) multi-grid method (100 iterations); (c) multi-grid method (1000 iterations); (d) multi-grid method (10,000 iterations); (e)–(h) the corresponding errors.
Fig. 4. Schematic diagram of the experimental setup. 1, laser; 2, 3, spatial filter and collimating lens; 4, phase object; 5, 6, Ronchi gratings; 7, 9, imaging lenses; 8, filter; 10, screen.
Fig. 5. Moiré fringes: (a) candle-air flame; (b) heated air around an electric iron; (c) alcohol lamp flame.
Fig. 6. Wrapped phases: (a) candle-air flame; (b) heated air around an electric iron; (c) alcohol lamp flame.
Fig. 7. True phases: (a) candle-air flame; (b) heated air around an electric iron; (c) alcohol lamp flame.
Fig. 8. True phases successively processed using the multi-grid method iterated 100, 1000, and 10,000 times, as well as the flood-fill method, respectively: (a1)–(a4) candle-air flame; (b1)–(b4) heated air around an electric iron; (c1)–(c4) alcohol lamp flame.
Fig. 9. Comparison of residuals under different numbers of iterations: (a) flood-fill method; (b) multi-grid method.
Fig. 10. Comparison of residuals under different threshold values: (a) different methods; (b) different flow fields.
Fig. 11. The deflection angle distributions of the 256th (central) row.
|
Table 1. Comparison of Accuracy and Time
|
Table 2. Comparison of Time
Set citation alerts for the article
Please enter your email address
© Copyright 2018-2021 | Chinese Laser Press. All Rights Reserved 沪ICP备15018463号-20