Shuo Li, Rong Zhang. Quantum Walk Wave-Particle Coherent Superposition[J]. Laser & Optoelectronics Progress, 2024, 61(5): 0527002
- Laser & Optoelectronics Progress
- Vol. 61, Issue 5, 0527002 (2024)
Fig. 1. Quantum walk circuit diagrams. (a) Wave quantum walk; (b) a left and right conditional walking operation; (c) particle quantum walk; (d) wave-particle quantum walk
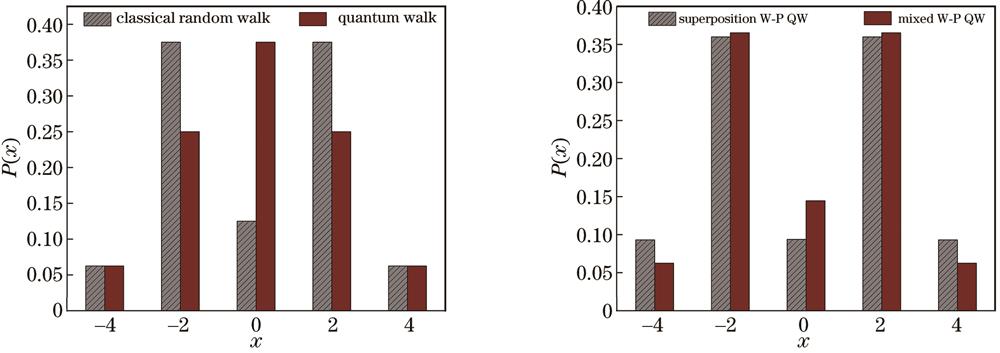
Fig. 2. Probability distribution calculated by even step position theory after 4-step evolution when
Fig. 3. Change of position variance with the theoretical calculation of step t when
Fig. 4. Changes of position variance with the theoretical calculation of auxiliary bit parameters. (a) When
Fig. 5. Wave-particle quantum walk demonstrated in IBM quantum computer
Fig. 6. Probability distribution of even step position simulation after 4-step evolution when
Fig. 7. Changes of position variance with the simulation of step t when
Fig. 8. Changes of position variance with the simulation of auxiliary bit parameters. (a) When
Fig. 9. 4-step evolutionary probability distribution after adding noise when
Set citation alerts for the article
Please enter your email address
© Copyright 2018-2021 | Chinese Laser Press. All Rights Reserved 沪ICP备15018463号-20