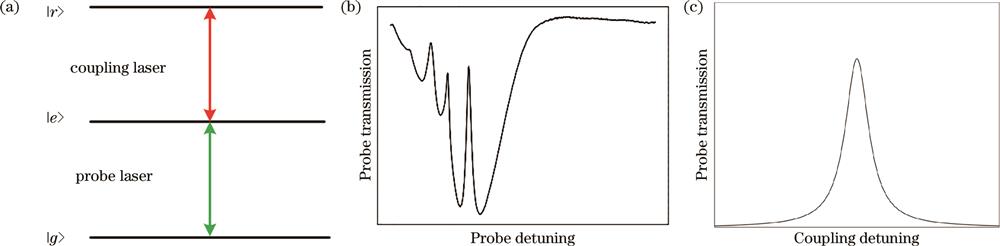
Yanzheng Wang, Bo Wu, Yunqi Fu, Qiang An. Investigation of Electromagnetically Induced Transparency Spectrum in Cesium Atomic Vapor Cell[J]. Laser & Optoelectronics Progress, 2025, 62(3): 0302001
Search by keywords or author
- Laser & Optoelectronics Progress
- Vol. 62, Issue 3, 0302001 (2025)
Abstract
Keywords
Set citation alerts for the article
Please enter your email address



