Ghost imaging (GI) captures images based on the spatial correlation of two separated beams. In the first ten years since its demonstration, the development of ghost imaging has mostly been driven by discussions on its fundamental principle, that is, whether or not ghost imaging is inherently a quantum effect. Initially, ghost imaging was achieved based on entangled photons^[1,2]. Advanced wave theory^[3,4] was proposed as a concise and clear phenomenological explanation of its principle. The first controversy about the principle of ghost imaging is whether entanglement is a necessary condition for ghost imaging^[5]. This problem was answered when classical correlated light sources were implemented to realize two-beam correlation imaging^[6]. In subsequent investigations, the discussion gradually shifted towards comparing the roles of entanglement and correlation in ghost imaging^[7–10]. A large amount of theoretical and experimental research was devoted to this stage, which gave birth to classical ghost imaging based on pseudo-thermal light^[11–13]. Since then, ghost imaging has begun to develop in two different directions. Quantum ghost imaging applies the quantum peculiarity of entangled light sources to the imaging domain and promotes imaging performance beyond traditional imaging methods^[14–19]. Classical ghost imaging has gradually evolved into computational ghost imaging^[20], and combined with computational technologies, such as compressed sensing and deep learning. It provides solutions for real-life applications^[20–23].

In the early stage, most ghost imaging research focused on pure amplitude objects. A milestone in ghost imaging is its combination with holography. The introduction of holography has propelled advancements in phase information acquisition within ghost imaging research. The extraction of phase information can be achieved through intensity correlation and interference techniques^[24-29]. Iterative algorithm^[30,31] and quantitative phase shift^[32] further enhance the capability for phase reconstruction. The idea of ghost holography is applied to quantum state measurement and becomes an effective method for recovering the quantum state^[33]. However, challenges persist in current quantum holography endeavors, including imperfect phase recovery, prolonged recovery time, and specific object requirements. Overall, quantum holography remains a promising avenue worthy of further investigation.

Finally, we review the implementation of single-photon sensitive cameras in ghost imaging. The application of the intensified charge-coupled device (ICCD) in ghost imaging^[34] enables the circumvention of the limitations imposed by point scanning, facilitating rapid and accurate capture of signal photons. Furthermore, owing to the quantum properties exhibited by entangled photon pairs, the requirements for light intensity^[15] and wavelength^[14,35,36] are further reduced. By virtue of these benefits, ghost imaging has emerged as a crucial tool for investigating and applying fundamental principles in quantum theory^[37–39]. In certain practical scenarios, combining image processing techniques, such as edge-enhanced imaging and optical image recognition, with ghost imaging often yields significant results with minimal effort^[40–42]. Consequently, the utilization of ICCD provides a substantial impetus and inspiration for advancing quantum imaging and related technologies.

In the next section, we review the origin and development of ghost imaging, especially in its early stages. Following that, we introduce recent advances in ghost imaging from two perspectives: ghost imaging in the realm of holography and the implementation of single-photon sensitive camera.

Ghost imaging is an imaging scheme based on the high-order spatial correlation of light sources. In a typical ghost imaging system, the object and spatially resolving detector are located in separate optical paths. Two spatially correlated beams illuminate the object and the spatially resolving detector, respectively. Light transmitted or reflected from the object is detected by a single-pixel detector. The other beam, which is normally called a reference beam, never interacts with the object but gets detected by the spatially resolving detector. Detection signals from either of the two detectors solely cannot recover the information of the object. In comparison, the image of the object can only be reconstructed by the joint measurement of two detectors.

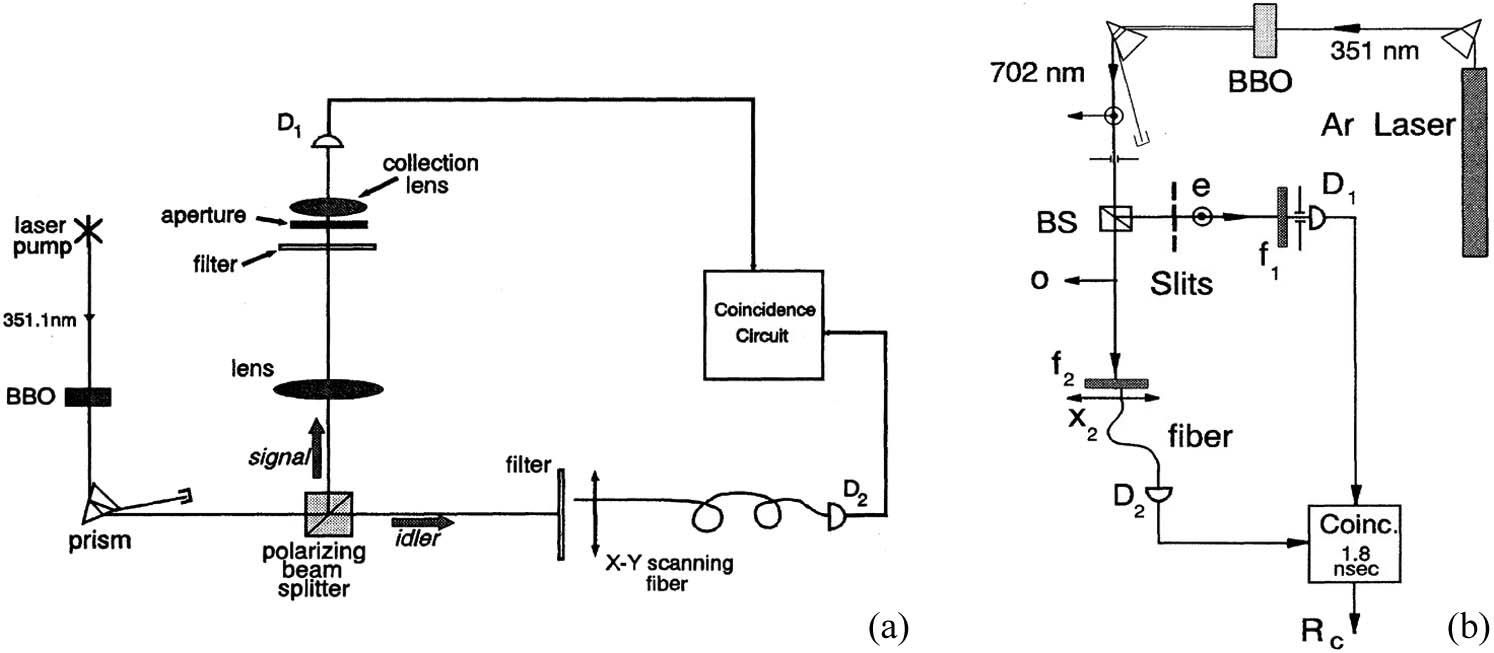
In the very beginning, ghost imaging was based on the spatial correlation of entangled photon pairs from spontaneous parametric down-conversion (SPDC), which is a nonlinear optical effect. In this process, a pump photon is incident on the nonlinear crystal and split with a certain probability into two down-conversion photons, denoted as the signal photon and the idler photon. The two down-conversion photons are entangled due to the conservative nature of this process. In 1995, ghost imaging was experimentally demonstrated for the first time^[1]. In this work, pairs of entangled photons are produced by using a laser with a central wavelength of 351.1 nm to pump a Type-II BBO crystal. Then, the two entangled photons are separated by a polarizing beam splitter. The signal photon probes a transmitted object and is then detected by a bucket detector, while the idler photon is directly incident on a position-scanning point detector, which acts as a spatially resolving detector. A schematic diagram of the experiment arrangement is shown in Fig. 1(a). The image of the object could be obtained by collecting the coincidence counts of these two detectors while recording the position information of the scanning one.

A phenomenological description of the relationship between the object and its image in quantum ghost imaging was given by Klyshko et al.^[3,4]. Their theory reveals how the relative position between the object and the detector influences the experimental results. They consider that the object detector could be seen as a light source in a traditional imaging system, and the nonlinear crystal would be regarded as a reflected mirror. The curvature of this mirror is related to the wavefront of the pump beam. So we can unfold the two-beam ghost imaging scheme to an equivalent classical imaging process to predict the result. The light leaves the object detector, passes through the object, and then is reflected by the nonlinear crystal. The reflected light keeps propagating forward to the spatially resolving detector. Finally, we could get an image of the object on this detector. This elaboration is often referred to as the advanced wave theory or the two-photon geometric optics. When the array detector is located in the far-field plane of the object and the object detector is changed into a point detector rather than a bucket detector, we can obtain the diffraction pattern of the object, which corresponds to the process of ghost diffraction. The ghost diffraction experiment^[2] was published in the same year ghost imaging was born. The experimental diagram is shown in Fig. 1(b).

Following the demonstration of ghost imaging with entangled photon pairs, a controversy comes up as to whether this novel imaging scheme is quantum inherent or if it can be reproduced with classical light sources. In 2001, a theoretical work claimed that entanglement is a prerequisite for ghost imaging. Classical light sources even with strong correlations cannot realize similar effects from two-beam correlation^[5]. This point of view soon attracted extensive attention. However, shortly afterward, a classical mimick of quantum ghost imaging was realized based on classical correlated light sources (Fig. 2)^[6]. A laser beam is used to generate an angle-dependent illumination between two beams and mimic the momentum entanglement of the SPDC photon pairs. The image of the object would be obtained only by the joint measurement of the two beams. Their work proves that ghost imaging does not require entanglement. Nevertheless, in Ref. [6], only the far field correlation detection is demonstrated, leaving an open question on whether classical correlation can reproduce strong correlation in both the near and far fields.

Inspired by the above two works^[5,6], more and more work has begun to explore the nature of ghost imaging. In 2003, a theoretical work^[7] showed that ghost imaging can also be achieved by obtaining second-order correlation functions for “classical” mixture states with correlation properties. At the same time, they also tried to point out the differences between the entangled state and the “classical” mixture state in ghost imaging. They considered that the “classical” mixture state can only realize one of the near field or far field spatial correlations, while the entangled state has spatial correlation in both the near field and the far field. This also raised important questions for subsequent theoretical and experimental work: what are the differences between the correlation and entangled light source in ghost imaging since classical light source can also achieve ghost imaging?

In 2004, two theoretical works proposed the feasibility of using pseudo-thermal light to produce classical correlated light and conducting ghost imaging experiments through second-order correlation of light fields^[10,11]. Pseudo-thermal light has high spatial correlation properties in both near and far fields, which are similar to the properties of entangled light sources. Ghost imaging and ghost diffraction can be realized under pseudo-thermal light illumination. Shortly after these theoretical works, ghost imaging based on pseudo-thermal light was experimentally achieved^[12,13]. The schematic diagram of the classical ghost imaging can be seen in Fig. 3. In the experiments, pseudo-thermal light was produced by a laser beam illuminating a piece of slowly rotating ground glass. A beam splitter was used to split the pseudo-thermal light into two beams to simulate the spatial correlation property of entangled photons. In addition, in 2005, Wu et al. realized ghost imaging with true thermal light for the first time^[43]. The light emitted from a hollow cathode lamp was used as the true thermal incoherent source. This work proved that true thermal light can also carry the correlation conditions required for ghost imaging.

In the above work, the spatial correlation of both near field and far field of classical light source is studied. These experiments realized ghost imaging and ghost diffraction based on classical correlated light sources, which were once thought to be surpassed only by entangled light sources^[13]. However, a pair of photons emitted by a classical correlation source lacks the property to violate the Heisenberg-like uncertainty relation of the difference of photons’ position and the sum of photons’ transverse momentum^[8,9,44], while entangled light sources can exceed this limit. Except for this discrepancy, ghost imaging of classical correlated light sources can be viewed as a complete mimic of quantum ghost imaging.

In the theoretical framework of the above work, ghost imaging can be regarded as a high-order correlation from either quantum entanglement or classical partial coherent fields. From then on, the high-order correlation has been widely discussed, the essence of which is precisely stated in Ref. [45]. In 2008, Cao et al. investigated the arbitrary Nth-order intensity correlation measurement with thermal light theoretically and experimentally^[46]. In the thermal light double-slit interference scheme, they demonstrated that the visibility and resolution of the interference pattern could be dramatically enhanced when the order N becomes larger. In the following studies, the geometric optics description of ghost imaging has become complete. It gives different models for entangled and classical correlated light sources^[47,48].

So far, the debate about the essence of ghost imaging has been basically resolved. In the subsequent development, entanglement-based and classical correlation-based ghost imaging has flourished in their respective directions. The former promotes the application of quantum superiority in the imaging field, such as wavelength conversion imaging^[14], superresolution imaging^[16,17], and sub-shot-noise imaging^[18,19]. Classical correlation-based ghost imaging has promoted the study of spatial high-order correlation in combination with information optics^[49–51]. It has given solutions to many practical problems^[52–54]. A possible application is classical ghost imaging in X-ray imaging^[11]. Thanks to the development of computational optics, classical correlation-based ghost imaging has evolved into single-pixel imaging. Later, single-pixel imaging was widely integrated with computer technology, such as compressed sensing, providing a new option for imaging applications in production and life^[20–23].

The imaging scheme in which two beams of light image an object by correlation measurement may be called ghost imaging, quantum imaging, or correlation imaging in different applications, and this article makes no distinction here.

Since the inception of the ghost imaging experiment, extensive research has been conducted on imaging the amplitude information of the object through second-order correlation using either entangled or classical correlated light sources. However, the phase information of the object is lost during this process. It is worth noting that phase information holds significant application value in various fields, such as biomedicine^[55], astronomy^[56], and information encryption^[57]. Therefore, a comprehensive investigation into quantum holography assumes paramount importance.

Research on ghost holography has lasted for more than 20 years. According to the fundamentals of Fourier optics, an object’s phase information can be presented in its diffraction pattern or interference fringes but not in the image. Inspired by this, ghost diffraction and interference were initially harnessed as means to derive phase information in early studies of ghost holography. Phase retrieval relies on the inverse Fourier transform of the obtained diffraction or interference pattern. The drawbacks of this scheme include its incapacity for quantitative phase information reconstruction and prolonged time consumption attributable to the iterative algorithm. In recent years, the methods of traditional holography, such as four-step phase shifting and digital holography, have been gradually integrated into ghost holography. This progress promotes quantitative phase retrieval of ghost holography to be accomplished efficiently.

In 2001, a theoretical framework was first proposed on the concept of quantum ghost holography^[24]. Building upon the conventional optical path of ghost imaging, the researchers employed a bucket detector to capture interference signals from the scattered light and the directly transmitted light of the object. The spatial correlation of entangled light sources was used to achieve non-local phase imaging. In 2004, by incorporating a phase double-slit into the quantum ghost diffraction optical path as an object, the same team^[24] successfully reconstructed its diffraction fringe through coincidence measurement^[25]. Their work demonstrated that ghost diffraction can convey phase information and holds the potential for phase recovery both theoretically and experimentally. However, this scheme is limited in its ability to quantitatively recover phase information without additional algorithms.

Concurrently, classical light sources have also made notable advancements in ghost holography. In 2006, Borghi et al. introduced the structure of Young’s interferometer in a ghost imaging setup for the first time, successfully conducting the first experiment on ghost holography based on pseudo-thermal light^[26]. The schematic diagram is shown in Fig. 4. This approach utilizes Young’s interferometer to capture the interference fringe between two points on the object’s Fourier plane through correlation measurement, as depicted in $$I_{\mathrm{tot}}(\mathcal{E})=I_1+I_2+2\sqrt{I_1{I}_2}|g|\cos (\frac{2\pi d}{\lambda f}\mathcal{E}+\varphi ).$$(1)

By calculating the contrast $|g|$ and fringe displacement $\varphi$, both amplitude and phase information of the spatial frequency spectrum can be obtained. The phase information can then be recovered through inverse Fourier transform. Compared to conventional methods for phase recovery in Young’s interferometer, this technique employs intensity correlation to acquire phase information, thereby only necessitating partially coherent light. This advantage is also shared by all ghost holography techniques. However, due to its limitation of obtaining phase information for only one point at a time, it requires much time to acquire high resolution phase information.

In order to mitigate the prolonged phase recovery time caused by the point scanning method, researchers promptly conceived utilizing a camera instead of a point detector. In 2009, Wang’s group proposed an approach to achieve interference and diffraction under incoherent illumination conditions^[27], as shown in Fig. 5. The laser illuminates the rotating ground glass to produce a pseudo-thermal light source that enters the Mach–Zehnder interferometer (MZI). The two arms of the interferometer are represented by the signal light $E_o$ and the reference light $E_r$, respectively. $E_o$ designates the arm where the object is located, and $E_r$ represents the other. Two CCD cameras are equipped at either end of the interferometer for measurement. Due to the incoherent nature of the illumination source, a clear diffraction image $\tilde{T}(\tilde{x})$ cannot be obtained directly by the CCDs. However, it can be obtained by the intensity interference method averaging out at multiple images acquired by the CCDs, depicted as $$⟨E_o^{*}(x)E_r(x)⟩=A\tilde{T}(kx/(2f_0)).$$(2)

Owing to a $\pi$ phase difference between ${\mathrm{BS}}_1$’s two exits, ${\mathrm{CCD}}_1$ captures an interference image of $E_o+E_r$, while ${\mathrm{CCD}}_2$ captures an image of $E_o-E_r$. By adding and subtracting these two images, interference component $\tilde{T}(\tilde{x})+\text{c.c.}$ and background component ${|\tilde{T}(\tilde{x})|}^2$ can be distinguished.

In 2010, Han’s group achieved the acquisition of non-local phase information by integrating a ghost imaging optical path on this foundation^[28]. Although this scheme successfully achieves ghost holography solely through path interference and intensity correlation, it falls short of accurately retrieving phase information due to the absence of introducing a complete phase shift.

In 2013, Wang’s group demonstrated the ghost holography of one-dimensional phase objects by harnessing the Talbot self-imaging effect of periodic objects in conjunction with an entangled source and coincidence measurement^[29]. This experiment realized quantum ghost holography through the spatial interference effect of two-photon wavepackets. However, it should be noted that perfect phase information was not obtained due to solely relying on the Talbot effect without introducing any additional phase shift.

With the development of ghost holography, researchers discovered that ghost holography could be employed to depict the information of the photon state. In 2016, Jachura et al. recorded a hologram of a single-photon (HSP)^[33]. Their core idea is shown in Fig. 6. The experiment was conducted using entangled photon pairs and the interference effect between different exit paths to obtain cosine values of the phase of the unknown photon state. By employing suitable iterative algorithms^[58], they successfully achieved complete phase recovery. This approach necessitates an iterative solution with limited precision and accuracy due to the absence of a complete phase shift.

In traditional imaging, we can use the Fourier ptychography (FP) technique^[30] to achieve phase imaging without any interferometer structure. In 2019, Padgett’s group achieved precise phase recovery at low photon numbers based on the spatial entangled and FP technique^[31]. They generated entangled photon pairs through the SPDC with multi-mode characteristics. One of the two entangled photons is reflected by a spatial light modulator (SLM) and then collected by a single-mode fiber (SMF) connected to a single-photon detector, the other probes the object and then is incident into the ICCD. The object, ICCD, and SLM are all placed in the far field of the nonlinear crystal while the facet of the SMF is placed in the near field of the nonlinear crystal. The ICCD is triggered by the single-photon detector to post-select the entangled photon pairs and capture coincidence images of the object. Meanwhile, the SLM displays blazed phase grating with different periods. It combines with the SMF to realize different illumination angles, which is indispensable for the FP technique. By using images with different illumination angles and the FP algorithm, they retrieved the amplitude and phase information of the object, as shown in Fig. 7.

The initial focus of ghost holography research was on utilizing diffractive light paths in conjunction with various interferometer structures to acquire phase information with entangled or classical correlated sources. However, due to incomplete phase shifts, only partial phase information could be extracted, necessitating the use of iterative algorithms for approximating the phase. With advancements in SLM and single photon cameras, researchers have started exploring methods to achieve quantitative ghost holography through specific phase shifts and optimized ghost imaging optical paths.

In 2021, Faccio’s group proposed a ghost holography scheme based on a polarization-entangled light source^[32]. The core idea of their work is to accurately extract the phase through the four-step phase shifting method. The experimental scheme is depicted in Fig. 8(a). The polarization-entangled two-photon state is generated by the SPDC. In the far field of the nonlinear crystal, the entangled state is ${\sum }_k({|V⟩}_k{|V⟩}_{-k}+{|H⟩}_k|H_{-k}⟩)$, where $k$ represents the transverse momentum of the photons. In their experiment, two SLMs are set in the optical path. Alice SLM is used as the phase object. Bob SLM provides reference phases to realize phase shifting. The two-photon state is transformed into ${\sum }_k({|V⟩}_k{|V⟩}_{-k}+e^{i{\varphi }_k}{|H⟩}_k|H_{-k}⟩)$ after transmitting through the SLMs. Both the object’s phase and the reference phase are included in ${\varphi }_k$. The key of the four-step phase shifting method is to form the interference between the light field carrying object phase and the reference phase. A 45° polarizer is mounted in front of the electron-multiplying charge-coupled device (EMCCD) to yield four interference fringes, as illustrated in Figs. 8(f)–8(i). The phase information can be reconstructed using $$\varphi =\arg [I_0-I_{\pi }+i(I_{\pi /2}-I_{3\pi /2})].$$(3)

It has been demonstrated that this approach enables super-resolution imaging without noise like random phases. The only limitation is that the object needs to be polarization-sensitive.

Except for pure optics methods, there have also been recent endeavors employing the principle of digital holography^[59] in ghost holography.

In 2020, Pu’s team modified the pseudo-thermal light-based ghost diffraction optical path and introduced a random reference beam to achieve off-axis interference^[60]. By correlating the images obtained by two cameras, they acquired an off-axis hologram of the object’s diffraction pattern. The amplitude and phase information were digitally reconstructed through off-axis holography. Therefore, all the details of the object could be completely recovered via inverse diffraction. This innovative approach reduces the reliance on phase shifts. Further, they used the spatial average count to enhance efficiency.

Zia et al. demonstrated the retrieval of two-photon states using digital holography technology^[61]. As shown in Fig. 9, the pump light is partitioned into two components. One serves as the signal light carrying unknown complex field information, and the other serves as reference light with a known state. These two beams are combined through a beam splitter and incident into a nonlinear crystal. A superposition of two biphoton states is generated. The off-axis hologram between these states can be obtained through coincidence measurement. The two-photon state pumped by the unknown field could be recovered via digital holography. This scheme visually retrieves the photon state by utilizing quantum state interference and digital holographic technology.

Ghost holography breaks the restriction of traditional interference. It facilitates the realization of non-local interference and further study of the properties of photon states. Benefiting from quantum light’s properties, acquiring better results with fewer photons becomes feasible in phase recovery. By moving the object out of the focus plane, Ortolanod et al. acquired a defocused image of the object through coincidence measurement based on the ghost imaging system^[62]. The quantitative phase recovery is achieved by employing the intensity transmission equation. Notably, this approach harnesses quantum multi-mode correlations to mitigate quantum noise effects in phase reconstruction and enables quantum-enhanced imaging.

Camphausen et al. proposed a quantum-enhanced wide-field phase imaging scheme that integrates a space-polarization hyperentangled state, a large field of view (FoV), a lensless interference microscope, and a single-photon avalanche diode (SPAD) array camera^[63]. The schematic diagram is shown in Fig. 10. They acquired the holography of both birefringent and non-birefringent phase objects by the four-step phase shifting method. The phase recovery of birefringent objects is realized by placing them between two Savarts shown in Fig. 10(b), while for non-birefringent objects, this is achieved by placing them outside the Savart shown in Fig. 10(c). Furthermore, leveraging the properties of $N00N$ states enables a sensitivity enhancement of $1/\sqrt{N}$. This quantum advantage gives the scheme great potential for photosensitive sample detection.

In addition to the above work, researchers have also utilized the high-dimensional entanglement and superposition properties of quantum states, among others, to realize some ghost holographic experiments that extend well beyond conventional methods, such as OAM holography^[64] using high-dimensional entanglement and undetected photon holography^[65]. In general, all the current ghost holography work more or less encounters some problems. For example, the phase recovery is inaccurate, the recovery process is time-consuming, and some experiments have specific requirements for objects. In brief, ghost holography still has significant room for improvement.

In the past decade, ICCD camera has significantly advanced ghost imaging, particularly in quantum ghost imaging. The biphoton produced by the SPDC is split into two paths. One of the biphoton is incident on the ICCD directly. The other passes through the object and is detected by a single-pixel detector. The output signals of the detector trigger the ICCD to capture the ghost image of the object. This image acquisition process can be equivalent to the coincidence measurement. A full FoV image could be obtained by the ICCD with only one shot, which can completely circumvent point scanning constraints and enhance imaging efficiency.

Aspden et al.^[34] pioneered the ICCD’s use in 2013 to conduct EPR ghost imaging experiments. As shown in Fig. 11, utilizing the SPDC process of a BBO crystal, a pump photon of 355 nm is converted into two entangled photons of 710 nm. The single-photon detector detects photons interacting with the object and triggers the ICCD to capture the corresponding entangled photons. There is an approximately 70 ns electronic delay of the ICCD triggering mechanism. To compensate for this, a free-space optical delay line of about 22 m is established to ensure that the detected two photons originate from the same entangled pairs. The delay line includes a series of $4f$ imaging systems in order to preserve spatial entanglement characteristics. Taking this proposal as a model, in 2015, Morris et al.^[15] demonstrated the application of quantum ghost imaging under conditions of extremely low light illumination. They reconstructed images of a wasp’s wing, which are displayed in Fig. 12. This work presents its application prospects in the field of biomedicine. Rubin et al.^[35,66] proposed the concept of dual-color ghost imaging and subsequently realized this experimentally^[36].

In 2015, Aspden et al.^[14] implemented a sparse photon microscopy technique with the utilization of an ICCD based on the dual-color ghost imaging scheme. They utilized 355 nm pump light to generate nondegenerate entangled photon pairs through a BBO crystal. Specifically, the idler photons are at infrared wavelength of 1550 nm while the signal photons are at 460 nm wavelength. They achieved imaging with visible light while employing invisible infrared light for object illumination. This conversion from invisible to visible light deftly overcomes the limitations of infrared single-photon sensitive cameras and paves the way for high-quality quantum imaging under mid-infrared illumination.

In 2020, Qiu et al.^[40] leveraged the multiple degrees of freedom of the photons (i.e., hyperentanglement state) and proposed a multi-channel parallel ghost imaging scheme. Based on this, in 2023, they realized the visualization of Hardy’s paradox via the contrast-to-noise ratio of the ghost images^[37]. In previous ghost imaging schemes, two photons sharing common past information, i.e., created from the same pump via the SPDC, are usually necessary. However, Qiu et al.^[38] realized the interaction-free quantum imaging, which does not meet that requirement. Their scheme is demonstrated in Fig. 13. Additionally, there have been several other attempts at using entanglement-swapped photons to achieve ghost imaging^[39].

Generally speaking, acquiring clear ghost images requires the accumulation of thousands of frames. However, certain applications, such as pattern recognition, only require the extraction of special features from images. With the aim of practical use, an interesting question arises naturally: how image processing can be achieved in quantum ghost imaging? Aspden et al.’s work^[41] demonstrated non-local edge-enhanced imaging of a pure phase object with minimal photons. The object is generated by an SLM, while the other SLM loads a holographic grating with orbital angular momentum (OAM) $l=1$ as a phase filter. This imaging system only requires 7300 photons for object identification, demonstrating significant practical value.

In 2019, Qiu et al.^[42] proposed a novel method of quantum pattern recognition by combining quantum ghost imaging and optical image recognition technologies through light field engineering. By incorporating the Fourier spectral information of a targeted human face into the pump light, they effectually modulate the correlation characteristics of entangled photon pairs produced by the SPDC, thus constructing an effective quantum analog of the VanderLugt filter (Fig. 14). In this scenario, when the idler photon illuminates the face, the corresponding signal photon would exhibit a pronounced correlation peak on the camera (Fig. 15). This recognition process occurs at the single-photon level without being perceivable to the sample. Therefore, this methodology holds great promise for advancing fields such as covert detection and non-destructive biological sample identification. Moreover, target recognition can be achieved quickly after accumulating only several frames.

This article first provides a comprehensive overview of the evolution of ghost imaging in the first decade after its birth. During this period, disputes over the essence of ghost imaging are the focus of research. Although originating in entanglement, it eventually turns out to be a classical behavior. Much of the theoretical and experimental work remains significant until now. They promote the development of ghost imaging and make a profound explanation for the higher-order coherence of light fields. Currently, with the progress of technology and detection instruments, ghost imaging has become an important approach to obtaining image information in some special scenes. This article focuses on two aspects of it: ghost holography and the application of single-photon sensitive cameras.

The introduction of holography provides new possibilities for obtaining phase information in ghost imaging. Although there are still some problems in the current work related to quantum holography, such as imperfect phase recovery, long recovery time, and certain requirements for objects, quantum holography is still a direction worth further research.

The application of ICCD cameras in ghost imaging plays an important role. They not only can capture photons quickly and accurately but also can achieve quantum imaging under extremely low light conditions. In addition, the application of ICCD cameras has expanded to dual-color ghost imaging schemes and the application of quantum ghost imaging in image processing, pattern recognition, and so on, providing important impetus and inspiration for the development of quantum imaging and related imaging technologies.

The essence of ghost imaging, ghost diffraction, and ghost holography is the usage of high-order correlation of light sources to complete the acquisition of images. This property of the light sources, especially the application of entangled sources, gives ghost schemes advantages over traditional schemes. First, the spatial resolution limit of traditional imaging schemes is inherently determined by the diffraction limit. In ghost imaging, the measurement of higher-order correlation makes the imaging an $N$-photon event. The imaging resolution can thus reach the standard quantum limit ($\frac{1}{\sqrt{N}}$ enhancement) or Heisenberg limit ($\frac{1}{N}$ enhancement), exceeding the Rayleigh diffraction limit in a traditional imaging system. In addition, sensitivity is an important indicator of imaging performance. High sensitivity requires efficiently capturing the image of a sample in the situation of low signal intensity. Under this constraint, compressing system noise is the key to obtaining an adequate signal-to-noise ratio (SNR). One of the important sources of noise is the shot noise of light. Shot noise is determined by the fluctuation of the photon number in time. The lowest limit of shot noise for classical imaging is reached by the coherent light, which obeys the Poisson statistics. For an entangled light source, the entangled photons are created simultaneously. The temporal fluctuation of the photon-number difference will verge to zero. Therefore, entanglement-based ghost imaging can exceed the limit of classical shot noise and realize sub-shot noise imaging. Such an imaging scheme is conducive to the acquisition of image information for light-sensitive or low-absorption samples. Additionally, the development of invisible light imaging will encounter constraints posed by the spectral response, quantum efficiency, and cost of array detectors. Owing to the distinctive characteristics of its light source, ghost imaging naturally emerges as an effective means to circumvent the spectral response limitation of array detectors and facilitate invisible light imaging. Entangled light sources can be generated through a nondegenerate SPDC process. Objects are illuminated by invisible photons, while visible photons are detected by an array detector. Ghost imaging allows simultaneous invisible illumination and spatially resolved detection of visible light. In the domain of quantum optics, ghost imaging serves as an effective approach to investigate and harness high-dimensional two-photon entanglement. High-dimensional entangled quantum systems exhibit better robustness and larger information capacity, and offer advantages in quantum mechanics research, such as the lower requirement for detection efficiency in the verification of Bell’s inequality. Currently, ghost imaging has accomplished photon wavefunction measurements and high-dimensional information transfer akin to quantum teleportation. In the future, it may offer feasible solutions for other high-dimensional quantum information technologies, such as quantum communication and quantum key distribution (QKD).

In summary, no matter whether it is classical ghost imaging or quantum ghost imaging, both are constantly pushing the development of imaging technology and providing us with more possibilities. In the future, we look forward to seeing more innovations and breakthroughs. We believe that our review will be of reference value for everyone to understand the development, history, current status, and application prospects of ghost imaging.